What The ACT Does

 There are many reasons why students find the mathematics on standardized tests difficult, but the one I’d like to focus on here––the phrasing of the questions––rarely gets mentioned. It turns out that a little knowledge about how the ACT constructs and words its questions can go a long way in helping students improve their ACT math scores.
There are many reasons why students find the mathematics on standardized tests difficult, but the one I’d like to focus on here––the phrasing of the questions––rarely gets mentioned. It turns out that a little knowledge about how the ACT constructs and words its questions can go a long way in helping students improve their ACT math scores.
When students learn math in school, many concepts are taught in only one way, and with very straightforward phrasing. One great example concerns something called the fundamental counting principle, which every student learns in about 5th or 6th grade. Here’s an example of a typical problem whose solution depends on this principle.
Jonathan goes to a restaurant whose menu features 3 options for the appetizer, 4 options for the main course, and 5 options for dessert. How many different meal combinations can Jonathan order?
- 9
- 36
- 60
- 108
- 180
We all started out tackling this type of problem by drawing tree diagrams, or sample spaces, to solve visually. Here’s what the diagram for this problem looks like (A = appetizer, M = main course, D = dessert).
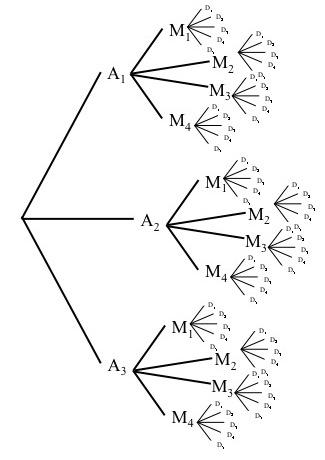
As soon as we realized this approach could get unreasonably tedious and time-consuming with even very small numbers, we learned the fundamental counting principle, which states that the total number of outcomes of independent events occurring together is simply the product (multiplication) of the number of possible outcomes for each event. In short, we can just multiply the total number of possibilities for each course, and we find that Jonathan has 60 possible meal combinations:
3 appetizers x 4 main courses x 5 desserts = 60 meal options
It’s fair to say that for math on a standardized test, it doesn’t get much easier. But it can get more difficult.
Here’s an example of how the ACT would phrase the same problem.
Jonathan goes to a restaurant and will order an appetizer for his first course, a main dish for his second course, and a dessert dish for his third course. Jonathan will choose 1 of the 3 appetizer dishes for his first course, 1 of the 4 main dishes for his second course, and 1 of the 5 dessert dishes for his third course. From among these 12 dishes, how many possibilities are there for Jonathan’s 3 dishes for the 3 courses?
- 9
- 36
- 60
- 108
- 180
Once again, this problem is conceptually identical to the first one presented above. I can guarantee, however, that if we gave the first problem to a random sample of 100 students, and the second to a random sample of another 100 students, the percentage of students who answered the second question correctly would be markedly lower. Let’s break down the major differences between the problems to see why.
(1) Wordiness. This is by far the most obvious difference. Including the numbers, the first problem––typical of what a student would see in school––was all of 32 words long. The second was about two-and-a-half times longer, at 81 words long. Word problems have always been reviled, much of the time unfairly so. But it’s certainly true that the  higher the word count, the greater the chance that students will be intimidated, will fail to comprehend, or will make a mistake. Some may argue that the extra language is necessary to specify that Jonathan orders only one dish for each course. While I’m willing to concede that this creates a more precise problem, there are very few students who wouldn’t already assume that as a given in the first problem’s phrasing (I bet you didn’t even think twice about it when considering it above!).
higher the word count, the greater the chance that students will be intimidated, will fail to comprehend, or will make a mistake. Some may argue that the extra language is necessary to specify that Jonathan orders only one dish for each course. While I’m willing to concede that this creates a more precise problem, there are very few students who wouldn’t already assume that as a given in the first problem’s phrasing (I bet you didn’t even think twice about it when considering it above!).
(2) Irrelevant numbers. This is a major pitfall for many students. In general, students learn in school that if the information is there, they’ll probably need it to solve the problem. Not so here. For example, the ’12’ is entirely unhelpful in solving. Even strong students will start to question themselves in this scenario: though they instinctively know that this number is extraneous, reaching a solution without it can be troublesome, and can lead students astray.
(3) Different language and presentation. Notice that in the second problem, there is no mention of “combinations” or “arrangements”. Furthermore, notice the phrases like “1 of the 5” and the incidence of “possibilities”, which students are trained to recognize as an indicator of fractions, percentages, and probabilities, but not combinations. These types of alterations can act as red herrings, leading students down time-wasting and often incorrect solution paths.
I don’t want to give the impression that I’m making a value judgment here: I do not consider the ACT’s phrasing “worse” or intentionally more confusing than the ones students typically see in school. In fact, it’s good to test for a well-developed ability to extract important information from rich context. As a leader of an organization that helps students address the challenges of exams like the ACT and SAT, what I do intend is to make clear that educators and students should recognize this element and how it can affect student score outcomes. Basic awareness of how problems are presented on all sections can make students more agile and capable test-takers––a goal everyone should be striving for.